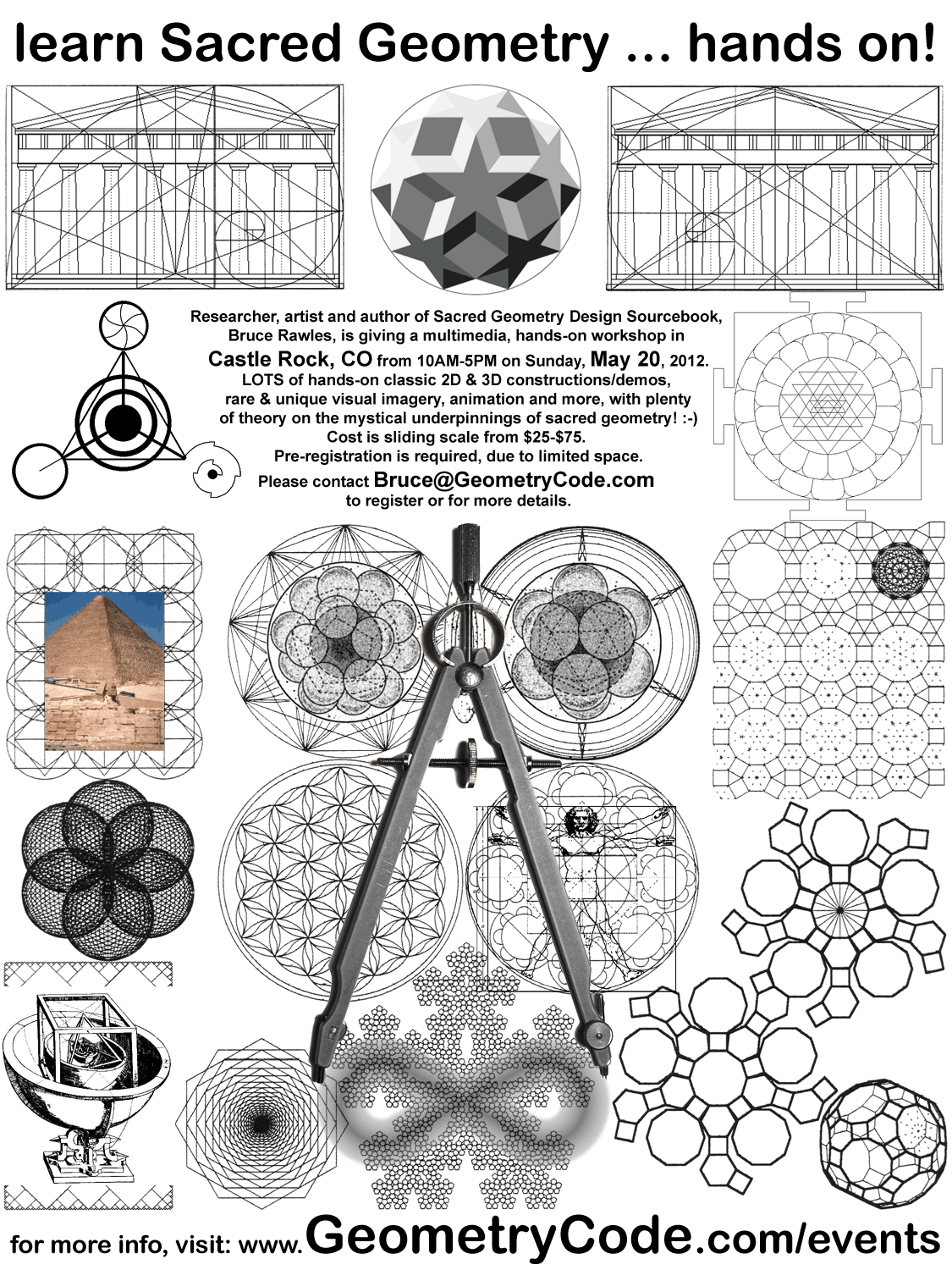
I’m giving a day-long, hands-on* sacred geometry workshop from 10AM-5PM this Summer 2012 (date TBA; rescheduled from May 20), here at our home in Castle Rock, Colorado. We’re about 30-60 minutes south of Denver, depending on what part of the metropolitan area you might be driving from. Cost is on a sliding scale from $25-$75 based on need. Please pre-register (required) due to limited space around our dining room table! :-) Since I limit the enrollment to a small handful, there is plenty of opportunity for individual Q&A, and exploring favorite geometries (and their metaphysical foundations) in an informal atmosphere. I always have days worth of material to cover if there’s interest, so no worries about that, either! :-) Please contact Bruce via GeometryCode.com to register. I’ll be happy to phone or Skype with even more details. Please bring a geometer’s compass, straight-edge, scissors and cellophane tape; I will supply lots of 2D and 3D handouts including platonic solid fold-up patterns, plus LOTS of visual multimedia imagery, animation and more, along with plenty of theory on the mystical underpinnings of sacred geometry! :-) I typically bring most of my extensive library on the subject out for everyone to peruse; you can see most of them (except some of the rare and obscure titles) on the GeometryCode Amazon aStore. Here is a full resolution printable flyer for the event.
Question from a form submission:
Q. I am a bit curious about geometry and was wondering if the workshop would be an appropriate place to learn more.
A. Definitely! I’ll be doing (and teaching) lots of hands-on demos of the classic Geometric constructions with compass and straightedge that folks like DaVinci, Plato, Pythagoras and more would likely have done, as well as LOTS of multimedia (books, animations, videos, stereograms, and more) demonstrating geometric principles that rarely (if ever) get covered in ordinary school curricula, blended with the mystical symbolism that the masters of both contemporary and ancient times revered and pondered. I have a flyer here that gives more examples and details, but feel free to ask me to cover specific topics since with a small group it’s easy to tailor it to the needs of those attending. Thanks for asking! :-) Note that the date shows May 20 on the flyer; but I’ve postponed it; I’ll update this page and the flyer when there’s a new date for the workshop. Please let me know ASAP if you’re interested, and which weekend dates work for you; also if you’d like an online class, let me know that too! :-)